Interference experiments with single photons:
Light as a wave or light as a particle?

In this lesson we want to investigate the behavior of photons in a device called interferometer. In the experiments discussed below, light shows wave and particle behavior in the same experiment. To explain these results, neither a pure wave model nor a pure particle model is sufficient. We further ask, whether a photon possesses the property "path" inside the interferometer: Does a particular photon take exactly one out of two possible paths from the source to the screen? The simulation experiment shows that we have to answer this question with no.


 
Inhalt:
1. Light in an interferometer
2. Interferometry with single photons
3. Does a photon go along a definite path?
    a) Polarisation filters in the interferometer arms
    b) Marking the photons
    c) Does a photon possess the property "path"?

1. Light in an interferometer

One of the most interesting and puzzling aspects of quantum mechanics is the duality of waves and particles. Photons and other quantum objects behave in certain experiments like waves and in other experiments like particles. In this lesson, we try to gain a deeper understanding of this mysterious quantum behavior.

A characteristic feature of waves is the appearance of interference. Let us therefore consider a device where interference can be demonstrated. It is called an interferometer.  Because the setup requires very precise adjustment which is not easy to achieve we use a computer simulation instead. The program can be downloaded here.

Experiment 1 (Computer simulation): The figure above shows a Mach-Zehnder interferometer. Light from a laser falls on a beam splitter (a half-silvered mirror). It is split into two beams that go along different paths (path A and path B). Mirrors deflect both beams deflected by 90°. Where the two beams meet, another beam splitter merges them into a single beam. 

Turn on the laser (click on the the button on the laser). On the screen, a pattern of concentric rings appears (Figure below).

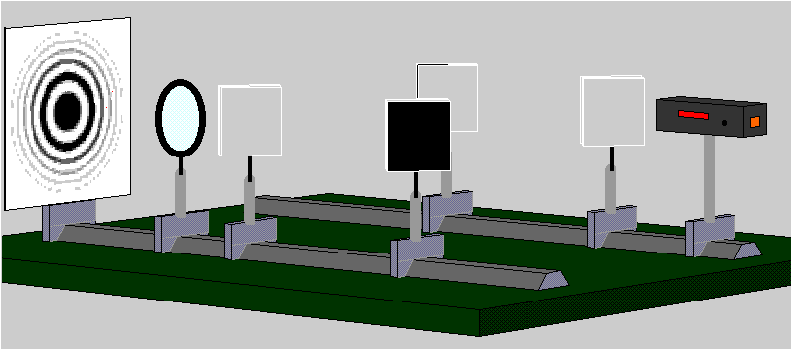
The essential point is that the light is split into two partial beams that go along different paths until they are merged again by the second beam splitter. The path differences along the different paths lead to an interference pattern on the screen.

Experiment 1 is an interference experiment, it demonstrates the wave properties of light. On the other hand, we know that this is not the whole truth. Light can also show particle properties. This led to the model as a stream of energy quanta called photons.

It would be tempting to carry out the interference experiment with single photons. What would happen then? Could we observe wave and particle properties of light in the same experiment? Would these two manners of behavior somehow coexist? Or do they exclude each other? We should try to perform the experiment to answer these interesting questions.


2 Interferometry with single photons

To perform experiments with single photons we need s source that emits very weak light. Only a few photons should leave the source at each moment of time. Similarly, we need very sensitive detectors to detect single photons. Both is indeed possible. As a source we use laser light that is attenuated by passing to several grey filters. To detect the photons special devices called CCD chip are employed (Details on the generation and detection of single photons).

Let us now perform the above interference experiment with single photons.

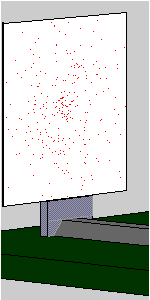
Experiment 2: In the simulation program, choose "single photons" at the source and switch the source on.

You will see that each photons excites (triggers?) only a single detector element on the CCD chip. The spatial pattern that results after the detection of a few photons is shown in the figure on the right. It seems to show no regularity.

When the number of detected photons increases you will see that from the "spots" of single photon detections a pattern gradually forms. You will recognize it as the circular interference pattern that has been observed in experiment 1 with laser light. If you click on the image to the right you will see how the pattern emerges gradually.

We found a similar result in the double-slit experiment considered in the previous lesson. In this experiment, a pattern formed out of the traces of single photons, too.

Experiment 2 shows - like the double-slit experiment - the duality of waves and particles for photons.Each photons transfers its whole energy to a single detector element. Such kind of a localized interaction is typical for particles.

In contrast, a wave is spread out over a whole region. A wave would spread its energy uniformly and would trigger a large number of detector elements.

Images

But the particle model alone does not suffice to explain the experiment either. The interference pattern that is formed by a lot of single-photon traces is a characteristic of a wave. It is not clear how in a particle model the formation of such a pattern could be explained. This illustrates clearly that in quantum mechanics, there is no simple alternative between waves and particles.

It is not possible to explain the physical behavior of light in a pure particle model or a pure wave model. A satisfactory description must contain aspects of both models.

3. Does a photon go along a definite path?

a) Polarization filters in the interferometer arms

The observation of  wave and particle behavior in the same experiment is quite peculiar on its own. But photons behave even stranger. When detected, a photon excites only a single detector element. Can we regard it as a similarly localized and particle-like entity inside the interferometer too?
If this were the case each photon had to take either path A or path B on its way to the detector. It had to choose one and only one of the two possibilities.

To answer this question we use the notion of a dynamical property that has been introduced in the preceding lesson (see also the basis page on preparation).

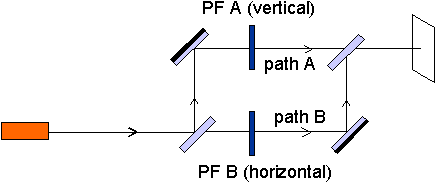
We ask whether  a photon inside the interferometer possesses the property "path". This is certainly the case when we assign a mark (tracer?) on each photon that allows to distinguish between path A and path B. To mark the photons we can e use their polarization.

What is the polarization of light?
 
Polarisation as a dynamical property

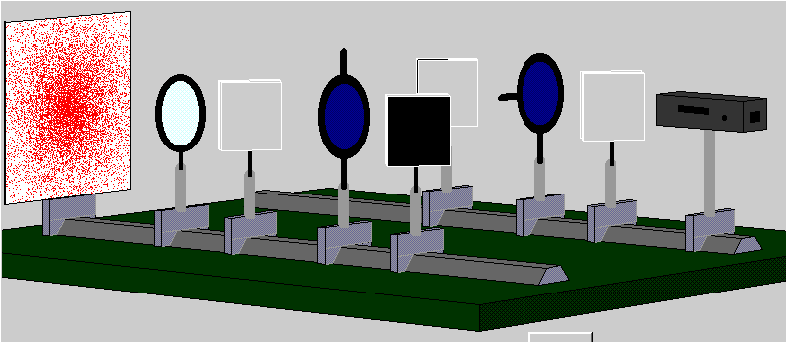
Let's do a preparatory experiment first:

Experiment 3: Click on the laboratory table. A window will appear where you can change the setup of the lab. Activate the polarization filters in each of the interferometer arms (figure). By dragging the levers on the polarization filters with the mouse you can adjust their directions. 

Adjust both levers vertically and switch on the source. You will notice that as in Experiment 2 the interference pattern forms out of many photon traces. We obtain the same result in we repeat the experiment with both polarization filters in horizontal position.

The polarization filters did not change the result of the experiment. The only notable difference is that the formation of the pattern takes a longer time. This is because the polarization filters absorb half of the photons. But there is one remarkable point: All photons that are detected on the screen have passed through a vertical polarization filter. They are therefore vertically polarized.

b) Marking the photons

Now we can turn to the interesting point. We mark with the polarization filters the paths A and B. To this end, bring the lever on polarization filter B in horizontal position. Leave polarization filter A in vertical position.
 
Each photon now carries a mark from which we can determine throgh which polarisation filter it passed.Why is this so? Let us ask what we could infer from detecting a vertically polarized photon on the screen. Obviously it must have come along path A. Path B is out because the polarisation filter there is in horizontal position and would not let a vertically polarized photon pass through. In this way, we can decide for each single photon whether it passed through polarization filter A or B. The property "path" is marked by polarisation.

Does the fact that we can say for each photon that it has chosen one particular of the two paths any consequences on the result of the experiment? Let's explore this question and carry out the experiment.

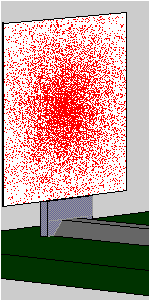Experiment 4 (Computer simulation): Turn the lever on polarisation filter B into horizontal position and switch on the source. Again, each photon excites only a single detector element. However, the traces of many detected photon do not form an interference pattern. You will observe a structureless distribution instead (Figure below). If you click on the image to the right you can see how this distribution gradually forms. 

c) Does a photon possess the property "path"?

Let us compare experiment 3 and experiment 4. Turning the lever in path B by 90 degrees was sufficient to prevent the formation of the interference pattern. The small but significant difference between the two experiments is that we can ascribe in experiment 4 to each photon the property "path". That means: We can tell with certainty that it took one and only one of the two paths.

From this we can infer conversely - and this is really astonishing: In the experiment without the polarisation filters, the photons did not possess the property path. We are not allowed to claim that a particular photon has taken exactly one of the two paths. Marking the photon's path prevents the interference pattern.

It plays no role that the polarisation of the photons is not measured in the detector. It is sufficient that the photons carry the path information to prevent interference.

How can we describe this experiment mathematically?

We can formulate the general statement:

In quantum mechanics, it is possible that a quantum object does not possess a certain property like "path A" or "path B".
You can visualize this in another way, too: In experiment 3 where the interference pattern was detected, some places on the screen remained completely free of photon traces. Photons were detected at these places in experiment 4, however. Let us imagine a photon as a localized object that travels along, say, path A. Then it has to "know" somehow about the adjustment of polarization filter B, because depending on this it must or must not avoid the empty spots. It is hard to imagine how the photon could acquire this "knowledge" (figure). The picture of the photon as a localized object that travels along a definite paths runs into severe difficulties here. Quantum mechanics tells us that we have to give up this picture.
 
Does the photon split? Does half of the photon run along each paht?

Do you have questions or comments? Send an e-mail to the disussion group: